
The following animation illustrates (or will illustrate once it is loaded, which may take a moment or three) the idea of pulsed inverted aerobraking:

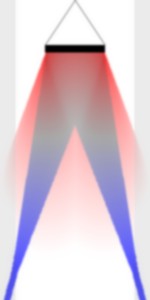
Figure 1: Schematic illustration of pulsed inverted aerobraking
Note that this figure is schematic. Especially the time dimension is distorted. Of course the animation is in slow motion. But furthermore the horizontal speed of the kinetic fuel (blue) may be exaggerated in comparison to its vertical speed. The kinetic fuel is released by passing robotic spacecrafts (small black buckets) and expands while it moves to the heat shield / pusher plate (big black structure) of the space ship (black triangle). After impact, the kinetic fuel (now red) expands vertically away from the pusher plate. Of course both the red and the blue density distribution varies in more complex ways than illustrated here. The expansion shape of the reflected plasma may be significantly different from this simple animation. A detailed physical simulation should be done.
Also any possible interaction of the reflected fuel with the next pulse of kinetic fuel is ignored. This interaction may turn out to be useful, e.g. by stretching the incoming cloud of kinetic propellant gas so that impact on the pusher plate takes longer and is therefore less shocking. Or the reflected plasma could be used to trigger release of the kinetic propellant more easy and/or realiable. On the other hand side if this interaction turns out to be harmful, the frequency of pulses must be reduced accordingly. Interaction can also be reduced by directing the reflected plasma away from the robotic swarm, e.g. towards earth. Directing reflected plasma towards earth helps to fight gravity during launch.
In this animation the kinetic fuel is delivered from two sides. In three dimensional reality of space, there are more possible directions to pass beside the space ship. Using more than one direction to place the kinetic fuel gives more possibilities to stabilize the space ship just by varying the time of release for the kinetic fuel. The kinetic fuel would impact more to one side or to the other side. Thereby the orientation of the space ship could be controlled actively, which in turn allows to control the position of the space ship in relation to the course of the robotic swarm. See also Notes on Stability. In case only two directions are chosen, they will most certainly not be in the same plane (as the animation might suggest); choosing them orthogonal might be a better idea.
Note furthermore that recoil due to releasing the kinetic fuel from the robotic space crafts may cause a significant change of course of the robotic space crafts. This is also ignored in the animation. Due to this change of course it would be better to deposit the kinetic fuel only from two sides, so it is easier for the robotic swarm to cluster together in two groups (plus possibly a group of robotic space crafts that did not release their fuel for some reason). These clusters could then be propelled and directed towards a rendevouz or the refilling point by a space tug which uses more efficient means of propulsion than the simple robotic space crafts can use (which must be simple to be economic, because there have to be a lot of them. See Size of Swarm).
Some people claim that Project Orion would have failed because its
shock absorber would "blow to smithers" (see Project
Orion). Tests with small (but not microscopically small) objects
did demonstrate that objects can stand short pulses of ulta-hot plasma
generated by atomic bombs, so one should think larger object should be
able to stand this too. I'm not an expert on this, so it may be true or
not. But even if this criticism is valid, it doesn't have to be valid
for Pulsed Inverted Aerobraking, which can be designed to work as
gentle as necessary so the shock absorber will stand a few minutes of
thrust.
One day in spring 2004, while I was walking along the seaside of
Tavemünde, I remembered that some years ago I was
driving a small and old pickup truck on an unpaved road in a desert.
That dirt road was shaped like corrugated iron.
When I first entered this road the car started to shake and tremble as
if it would fall apart the next moment.
I stopped. Realizing we had to go this way, I started again, slow. It
trembled again immediately, but the car
didn't fall apart. With that slow speed, it would take us forever to go
anywhere, so I dared to speed up a little.
Guess what: the shaking did not become worse. So I drove even faster
and to my big surprise, the
ride became much smoother somewhere between 60 and 90 km/h. It was
still bumpy, but the car's shock
absorbers took it quite well during the hours we travelled on.
Later I learned that this is a very common situation for off-road
driving. I did even see a slow-motion
video of a car travelling on terrain like this. The wheels do not drive
on the dirt, they jump from one
peak to the next, second-next or third next bump, being in the air most
of the time.
Remembering this I realized that it is a very similar situation to
Pulsed Inverted Aerobraking, from the mechanical
point of view. The car moves at around 10 to 20 m/s while the bumps are
10 to 20 cm wide.
So we get a bump frequency of about 100 per second. The time ratio of
contact to ground
to flying through air is something like 1:2. For Pulsed Inverted
Aerobraking at 100 Hz, fuel speed 10 km/s,
pusher plate and fuel cloud size 10 m, we get a ratio of 1:4.
The car has to stand an acceleration of 1 g. The space ship doing
inverted aerobraking has to stand about 3 g.
So Pulsed Inverted Aerobraking is not much more demanding than cruising
a desert track.
If an old pickup truck can do it, an affordable high tech space ship
should be able to do it too.
And with Pulsed Inverted Aerobraking you don't need tires that roll, so
the impact can be distributed over a much larger area. Contact area
between pickup truck and desert ground (the four tyres) is maybe 0.2
square meters, which is only something like 1/50th of the total area of
the pickup trucks bottom. So it becomes 50 times easier.
Of course there is a BIG difference: while the desert ground is
maybe 300 K hot,
the kinetic fuel has an effective
temperature of several 10000 K. (So
rubber tyres are not an option.) But heat shields have been built that
can stand this kind of heat for normal aerobraking, and as Project Orion tells us, with pulsed heat
things become even easier.
One may still say that the problem is not impulse but shock
velocity. Maybe the impact speed of the kinetic propellant is so fast
that it dissolves any solid substance not only by ablation (which was
the major concern in Project Orion research and is considered to be
solvable) but also from sheer shock.
But then we have to consider that the gas compressed in front of the
pusher plate is something like a one side open shock absorber too.
Maybe this layer builds up fast enough from the kinetic propellant
alone. Any incoming gas that is stopped by this mechanism adds to the
density of the gas layer.
So even if we don't have a gas layer there in the beginning of the
pulse, it should build up pretty
fast (at the cost of some ablation during creation of the layer).
And if it does not build up fast enough, we are free to add some
gaseous on-board fuel which expands away from the pusher plate the
moment before kinetic fuel arrives and builds up the gas cushion. (See On Board Fuel.)